The Nature of Periodic Points

Letform a– cycle of an analytic functionThen

1. 

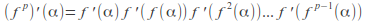
2. The derivative of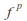takes the same value at each point of the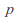– cycle so tha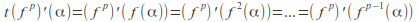

Proof

Sincewhere the functionis applied p times, we can deduce using the Chain Rule that

Puttinggives 1 above.

is the product of the derivatives ofat the points of the – cycle sois also the product of the derivatives ofat the points of the– cycle and similarly for the other points

The derivative ofat the point of a– cycle determines the nature of the point. Part 2 above implies that all the points of a- cycle have the same nature and we can talk of the nature of the– cycle as a whole.

Ifis a periodic point with periodof an analytic functionthenand the corresponding– cycle are

attracting if

repelling if

indifferent if

super attracting if

Example: Ifthen the period 2 – points areand

sand

soand the 2 – cycle consisting of the pointsandis repelling.