The second order, non constant coefficient, differential equation
with boundary condition
is finite is known as Legendre's equation. Solutions exist for
and the normalized solutions,
are polynomials of degree
known as Legendre polynomials. These polynomials are defined only on the interval
because at
or 1 the coefficient of
is zero.
The first few Legendre polynomials are given by
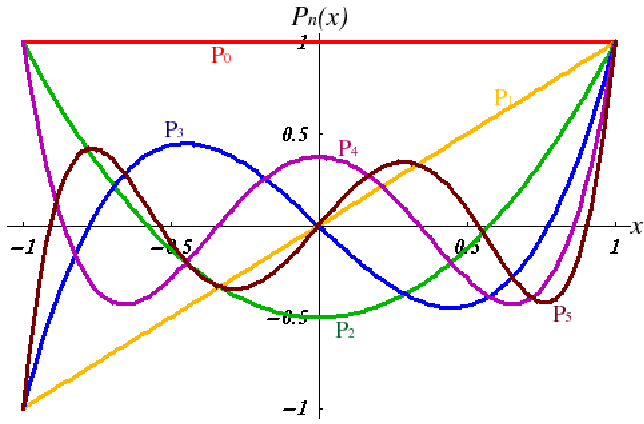
Legendre polynomials form a mutually orthonormal set, that is
where 
Proof: The P-n (x) satisfy
We can write this as
(1)
Similarly, for a solution
we can write
(2)
Multiply (1) byand integrate between -1 and 1:
Integrating the first term by parts gives
The first term on the right is zero:
Similarly for (2)
The right hand side of both equations are the same so
Since
we must have both sides are zero hence