Example:
\[\int_V 4xy^2 dV\]
over the volume bounded by the coordinate planes and the plane \[x+3y+2z=6\]
For the region shown,
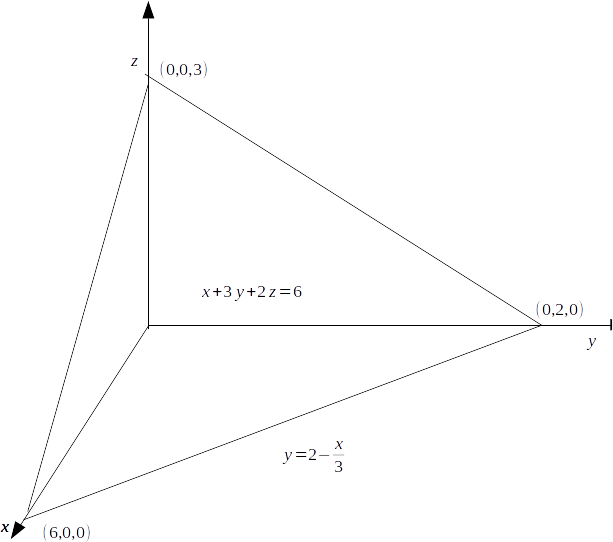
\[0 \leq z \leq 3 -\frac{x}{2}- \frac{3y}{2}\]
\[0 \leq y \leq 2 - \frac{x}{3}\]
\[0 \leq x \leq 6\]
This is not the only possible option. The integral becomes
\[\begin{equation} \begin{aligned} \int^6_0 \int^{2 - \frac{1}{3} x}_0 \int^{3 -\frac{x}{2}- \frac{3y}{2}}_0 4x y^2 dz dy dx &= \int^6_0 \int^{ 2 - \frac{x}{3}}_0 [4x y^2 z]^{{3 -\frac{x}{2}- \frac{3y}{2}}}_0 dy dx \\ &= \int^6_0 \int^{ 2 - \frac{x}{3}}_0 4x y^2(3 -\frac{x}{2}- \frac{3y}{2}) dy dx \\ &= \int^6_0 \int^{2 - \frac{x}{3}}_0 12x y^2-2x^2 y^2- 6xy^3 dy dx \\ &= \int^6_0 [4xy^3 -\frac{2x^2x^3}{3}- \frac{3xy^4}{2}]^{2- \frac{x}{3}}_0 dx \\ &= \int^6_0 \frac{x}{2}(2- \frac{x}{3})^4 dx \\ &= \frac{48}{5} \end{aligned} \end{equation}\]
