Proof That Any Element Not in a Compact Subset of a Hausdorff Space is in an Open Set That Has No Intersection With an Open Set Containing the Compact Subset

Theorem

Letbe a compact subset of a Hausdorff spaceIf a in X-A then open sets U and V exist such thatand

Proof

ChooseSinceand sinceis Hausdorff, open setsand exist such thatand

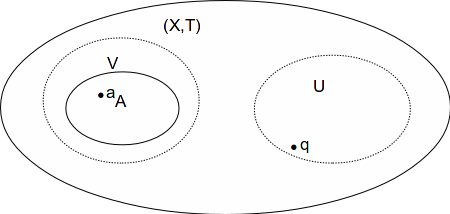
Since A is compact, a finite subcoverexists such that

Defineand

andare open, andand

But sinceforwe have