Theorem
Connectedness is a topological property. Equivalently, if![]() and
and![]() are topological spaces and
are topological spaces and![]() is continuous, then if
is continuous, then if![]() is (dis)connected then
is (dis)connected then![]() is (dis)connected and vice versa.
is (dis)connected and vice versa.
Proof
Suppose![]() and
and![]() are topological spaces and
are topological spaces and![]() is continuous. Suppose
is continuous. Suppose![]() is connected and Y is not connected. Then there are open sets
is connected and Y is not connected. Then there are open sets![]() such that
such that![]()
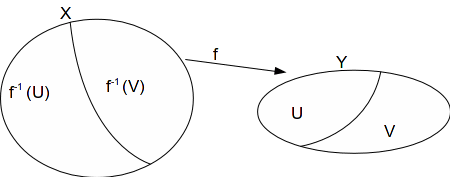
Since![]() is continuous,
is continuous,![]() are open sets in
are open sets in![]()
Since![]() is a bijection,
is a bijection,![]() and
and![]()
Hence![]() is disconnected - a contradiction. Hence
is disconnected - a contradiction. Hence![]() is connected.
is connected.
The other implications are very similar.
