The Basis For a Topology

A basis B for a topological space X with topology T is a collection of open sets in T such that every open set in T can be written as a union of elements of B. The base 'generates' the topology T. Also

1. The basis elements cover X.

2. Let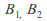be base elements and let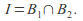For each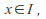there is a basis element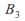containing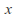and contained in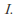By induction the union of any finite number of basis elements is also a member of the basis.

If a collection B of subsets of X fails to satisfy either of these, then it is not a base for any topology on X. (It is a subbase, however, as is any collection of subsets of X.) Conversely, if B satisfies both of the conditions 1 and 2, then there is a unique topology on X for which B is a base; it is called the topology generated by B. (This topology is the intersection of all topologies on X containing B.) A sufficient but not necessary condition for B to generate a topology on X is that B is closed under intersections.

For example, the collection of all open intervals in the real line forms a basis for a topology on the real line because the intersection of any two open intervals is itself an open interval or empty. In fact they are a base for the standard topology on the real numbers.

A base is not unique - more than one basis may generate the same topology, even if one basis is a subset of another. For example, the open intervalsis a basis for the normal topology onas is the set of open intervalsbut these two sets are completely disjoint and both properly contained in the base of all open intervals. A base need not be maximal, but a maximal base exists, given by the topology itself. In fact, any open sets in the space generated by a base may be added to the base without changing the topology.

The set S of all open intervals is a basis forbut for example, the set of all semi-infinite intervals of the formsandThen S is not a base for any topology on sinceis not a semi infinitte interval. One the other hand all semi infinite intervals of the formdoes form a basis forThe intersection of any finite number of the sets is just the smallest set, and of course2. is obviously satisfied.