The principle of least action is a variational principle that can be used to obtain the equations of motion for that system in a way that is independent of Newtonian mechanics.
The action principle is preceded by earlier ideas in surveying and optics. The rope stretchers of ancient Egypt stretched corded ropes between two points to measure the path which minimized the distance of separation, emphasized that one must correct for "deviations from a straight course"; in ancient Greece Euclid for light reflected from a mirror, the angle of incidence equals the angle of reflection; and Hero of Alexandria later showed that this path was the shortest length and least time.
Action is an attribute of the dynamics of a physical system. It is a mathematical functional which takes the trajectory of the system as its argument and returns a real number. Action has the units of Js and takes different values for different paths. Classical mechanics postulates that the path actually followed by a physical system is that for which the action is minimized, allowing the equation of motion of the system to be derived. The principle extends to quantum mechanics in the Feynman path integral formulation, where a physical system follows simultaneously all possible paths with amplitudes determined by the action. It also provides a basis for the development of string theory.
If the action is represented as an integral over time, taken along the path of the system between the initial time
and the final time
of the development of the system,
called the Lagrangian and is usually written L=T-V where T is the kinetic energy and V is the potential energy.
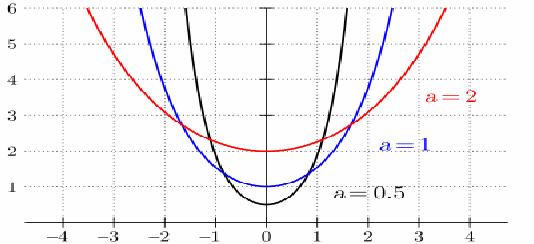
Probably the most famous example of the application of the action principle in mechanics is to the problem – if a length of rope acted on only by it's own weight is hung between two point, what curve it it describe? The curve, with equation
called a caternary and is shown below for various values of
