A system influenced by time dependent forces or which is represented in a rotating or non inertial reference frame has a Hamiltonian which depends explicitly on time,
The rate of change of the Hamiltonian is given by
On using Hamilton's equations of motion this becomes
The value of the Hamiltonian is not conserved, however the area is.
Proof:
and
not necessarily Hamiltonian. At time
the area of a region of phase space is given by
and at
Asincreases fromto
a point
inis sent to a point
in
so thatcan be considered as the initial condition on a trajectory
where
and the transformation fromto
is given by
The area at timeis given by
where 
is the Jacobian matrix of the transformation.
We can expand
and
in Taylor series:
Then the Jacobian can be written as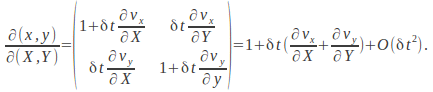
Consider
Hence
on use of Hamilton's equations and area is preserved.