Limit Cycles

For first order systems the motion tends to fixed points or infinity, but for second order systems the situation can be more complex. Consider for example a system separable in polar coordinatessuch that the motion has a fixed point (in terms of) other than at the origin:

whereare constant.

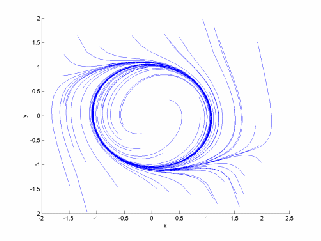
The circleis a phase curve and since the motion moves around it indefinitely it is a cycle. Any closed phase curve is a cycle – a motion for whichare periodic with the same period.

Forthe motion of the system above is a circle and we may treatas a fixed point of the system. In the region ofthe motion obeys the equations

where

The solution of this linearisation isand the phase curves are given by eliminatingfrom these two equations, obtaining

Ifthe system is stable in the sense that motion initially close to the system will be attracted to it, and any perturbed motion initially on the circle will return to it. Ifthe system is unstable.

A more general form of limit cycle is shown below with various starting points.