The Lennard-Jones or L-J potential is a mathematically simple model that describes the interaction between a pair of neutral atoms or molecules. The expression of the L-J potential is
where
is the depth of the potential well,
is the (finite) distance at which the inter-particle potential is zero, and
is the distance between the particles.
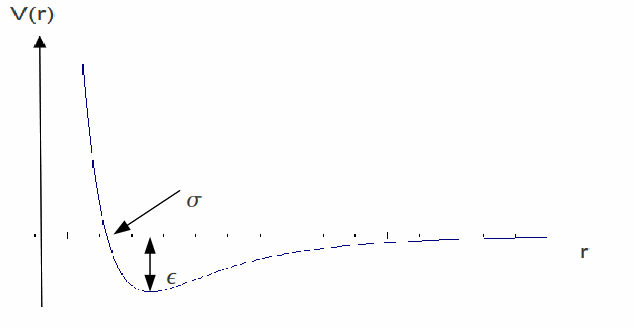
These parameters can set to reproduce experimental data or accurate quantum calculations. The
term describes Pauli repulsion at short ranges due to overlapping electron orbitals and the
term describes attraction at long ranges (van der Waals force, or dispersion force).The Lennard-Jones potential is an approximation. The form of the repulsion term has no theoretical justification; the repulsion force should depend exponentially on the distance, but the repulsion term of the L-J formula is more convenient due to the ease and efficiency of computing
as the square of
Its physical origin is related to the Pauli principle: when the electronic clouds surrounding the atoms start to overlap, the energy of the system increases abruptly. The exponent 12 was chosen exclusively because of ease of computation.The attractive long-range potential, however, is derived from dispersion interactions. The L-J potential is a relatively good approximation despite the arbitrary nature of the model and because of this and the model's simplicity is is used often. It is particularly accurate for noble gas atoms and is a good approximation at long and short distances for neutral atoms and molecules. Near the minimum of potential the graph of
may be approximated by a simple harmonic oscillator potential, which simplifies many calculations.
Differentiation ofgives
and setting this equal to zero allows us to findcorresponding to the minimum value for
