Problem solving may involve interpreting a real situation, representing it mathematically and finding an equation to solve. Other problems may involve fractions, percentages, ratios and probabilities all mixed up in so many different ways. Whatever the problem, you have to start by understanding it. Because of the variety of questions that can be asked, the process of answering them is best illustrated with examples.
Example: If I have £20 and I spend one quarter of my money on a pizza and one third of the remainder on a train fare, how much have I got left?
One quarter of £20 is £20/4=£5. After spending £5 of my £20 on a pizza I have £15 left. I spend one third of this, equal to £15/3=£5 on a train fare. This leaves me with £10.
Example: In 4 years time John will be three times as old as he is now. How old is John?
If John is
years old now, in 4 years time he will be
years old. The question says he will be three times older ie
so we have
John is 2 years old.
Example: Three people – Alf, Bob and Cal – have £450 to share. Alf gets twice as much as Bob but one third as much as Cal. Find how much each person gets.
Alf gets twice as much as Bob so if Bob gets £x, Alf will get £2x
Alf gets one third as much as Cal so Cal gets three times as much as Alf. If Alf gets £2x, Cal will get £6x.
The money is shared in the proportion 2x:x:6x which cancels down to 2:1:6. We now share £450 in the ratio 2:1:6. 2+1+6=9 so £450/9=£50.
Alf gets 2*£50=£100
Bob gets 1*£50=£50
Cal gets 6*£50=£300
How many of the small cuboid can fit inside the large one?
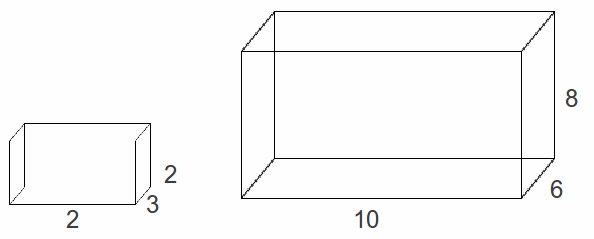
The small cuboids can be laid 5 along (5*2=10), 2 deep (2*3=6) and 4 high (4*2=8), so 5*2*4=40 small cuboids can fit inside the big one.