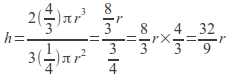If the volumes of two solid is in a certain ratio, we can often find relationships between some lengths of the solids even if the solids are not similar.
Suppose the volume of the cylinder and sphere below are in the ratio 2:3. The radius of the sphere is twice the radius of the cylinder, or equivalently, the radius of the cylinder is half the radius of the sphere. If the radius of the sphere is
find the height of the cylinder in terms of
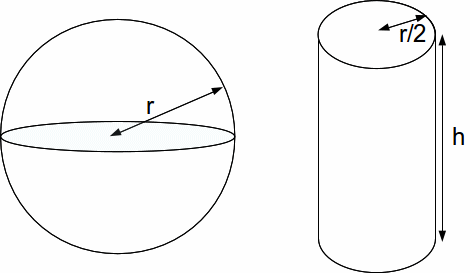
The volume of the sphere is
The volume of a cylinder of radius
and height
is
so the volume of the cylinder above is
The ratio given in the question means that
so
Then