As a race we need the most from our limited resources. Every manager must decide what or who to send where so as to get the job done with the least effort and risk, and at the least cost, or decide what products to make and sell, subject to the available labour and machine time, so that the profit will be maximised. The simplest sort of problem of this type is maximising an area subject to a fixed perimeter or maximising a volume subject to a fixed surface area.
Example: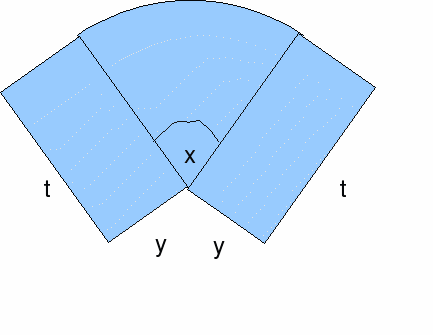
The perimeter of the shape is 100. x is 45 degrees.
Find expressions for the area and perimeter.
Find the value of x that maximises the area.
The perimeter is
which equals 100 from the question hence
(1)
We can rearrange the expression (1) to make y the subject:
Substitute this expression for y into the expression for the Area:
Since
is a square number >0, the maximum possible value for the area is 625, and the area takes this value when t=25.