We can prove that the sequence
is monotone increasing and bounded. Therefore it has a limit. The limit is in fact
We can use the binomial theorem to write
if
henceis bounded above by 3
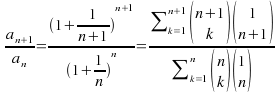
The kth term in the numerator is
The kth term in the denominator is
We must prove
then
and sequence is increasing.
The left hand side is greater than or equal to
but the left hand side is less than 3, so
is increasing and bounded, hence converges.
To find the limit, take natural logs:
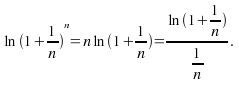
As
both numerator and denominator tend to zero so use L'Hopital's Rule.
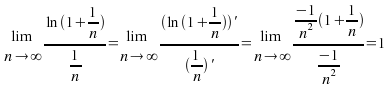
Exponentiation now gives
as the limit of