Roots and Convergence of Power Series

We can express convergence or divergence of a power seriesin terms of the nth root of the nth term.

Theorem

Letbe a power series.

1. If the sequence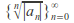is unbounded then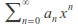converges only for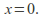

2. If the sequence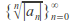converges to zero, then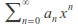converges for all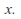

3. If the sequence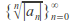is bounded and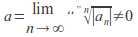then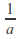is the radius of convergence.

Proof

1. Choose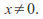For each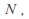there is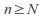such that 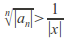hence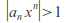for some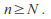In particular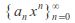does not converge to zero for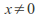hence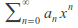diverges for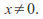

2. Suppose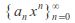converges to zero and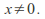Define 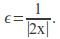There is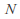such that for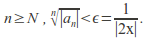Thus for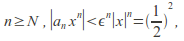so by the comparison test,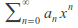converges for all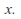

3. Suppose that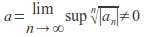and consider any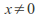such that 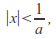then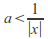so there exist real numbers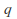and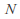such that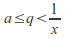and for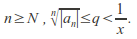Hence for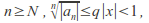therefore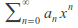converges absolutely. To show that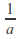is the radius of convergence, we must show that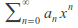diverges for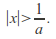Suppose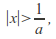then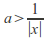hence there are infinitely many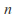such that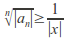so for infinitely many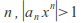so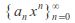does not converge to zero and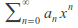diverges.