Let![]() be the generalized circle with equation
be the generalized circle with equation![]() with
with![]() and
and![]()
If![]() then
then![]() is the circle with centre
is the circle with centre![]() and radius
and radius![]() where
where![]() and
and![]()
Also![]() lies on the line through
lies on the line through![]() and
and ![]() and
and![]()
If![]() then
then![]() is the line through
is the line through![]() perpendicular to
perpendicular to![]()
Proof
Let![]() be the extended mobius transformation defined by
be the extended mobius transformation defined by![]()
![]() maps the circle defined by
maps the circle defined by![]() to the unit circle and so
to the unit circle and so![]() maps the unit circle to
maps the unit circle to![]() so that
so that![]() and
and![]()
Since![]() points on the extended real axis are mapped by
points on the extended real axis are mapped by![]() to points on the extended line
to points on the extended line![]() through
through![]() and
and![]()
If![]() then the diametrically opposite points -1 and 1 are mapped by
then the diametrically opposite points -1 and 1 are mapped by![]() to the diametrically opposite points
to the diametrically opposite points![]() and
and![]() of
of![]() on
on![]() because
because![]() is conformal at 1 and -1.
is conformal at 1 and -1.
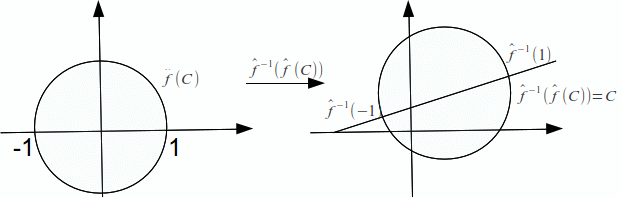
It follows that![]() has centre
has centre![]() on
on![]() given by
given by![]() and radius
and radius![]() given by
given by![]()
Furthermore![]()
![]()
If![]() then
then![]() so
so![]() must be a line that meets
must be a line that meets![]() in a right angle at
in a right angle at![]()
