The Radius of Convergence Theorem

For a given power series,precisely one of the following may happen

1. the series converges only for

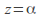
2. the series converges for all

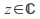
3. there is some real number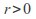such that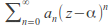converges and converges absolutely if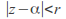and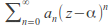diverges if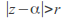

Example: find the radius of convergence of the sequence

in this example. The ratio test demandsfor convergence so

Assoand asthe right hand side tends to 0 so the sequence converges only forThe radius of convergence is 0.

Example: find the radius of convergence of the sequence

The ratio test demandsfor convergence so

Asfor allso the sequence converges for all

Example: find the radius of convergence of the sequence

The ratio test demandsfor convergence so

The radius of convergence is 1.