The Riemann mapping theorem states that if
is a non-empty simply connected region in
and
then there exists a bijective and holomorphic function
fromonto the open unit disk
The condition thatbe simply connected means thatdoes not contain any “holes”. The fact that f is bijective and holomorphic implies that it is a conformal map so preserves angles between lines or vectors and also the shape of any sufficiently small figure, possibly rotating and scaling it.
Poincaré proved that the functionis unique: if
and
is an arbitrary angle, then there exists a uniqueas above with the additional properties that
and that
This is an easy consequence of the Schwarz lemma.
As a corollary, any simple, connected subsets ofcan be mapped to each other because given
there exists a bijective holomorphic functionmappingto
and given
there exists a bijective holomorphic function
mappingtothen sinceis bijective,
exists and
mapsto
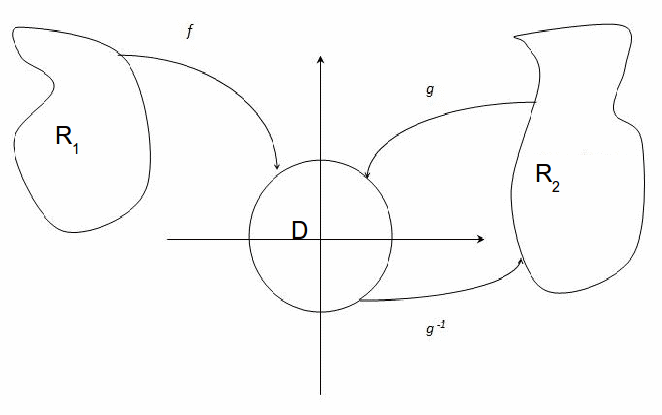
Further, since there exists a bijective mapping from the Riemann sphere onto
any two simply connected regions of the Riemann sphere can be mapped to each other.