Green's theorem gives the relationship between a line integral around a simple closed curve
and a double integral over the plane region
bounded by
It is the two-dimensional special case of the more general Stokes' theorem, and is named after British mathematician George Green.
Letbe a positively oriented (counterclockwise), piecewise smooth, simple closed curve in the plane
and let be the region bounded byIf
and
are functions of
defined on an open region containingand have continuous partial derivatives there, then
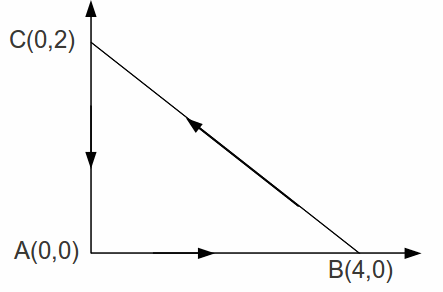
For example letbe the triangular region illustrated below and let
We need to find the limits. If we integrate with respect to
first then we must findas a function of
on the line BC:
Our integral becomes
Expanding the integrand and simplifying gives
