\[\oint_C P \: dx + Q \: dy = \int_R \frac{\partial Q}{\partial x} - \frac{\partial P}{\partial y} dx \: dy \]
The contour below \[C\]
is made up of the three paths \[C_1 , \: C_2 , \: C_3\]
which form the sides of the triangle.
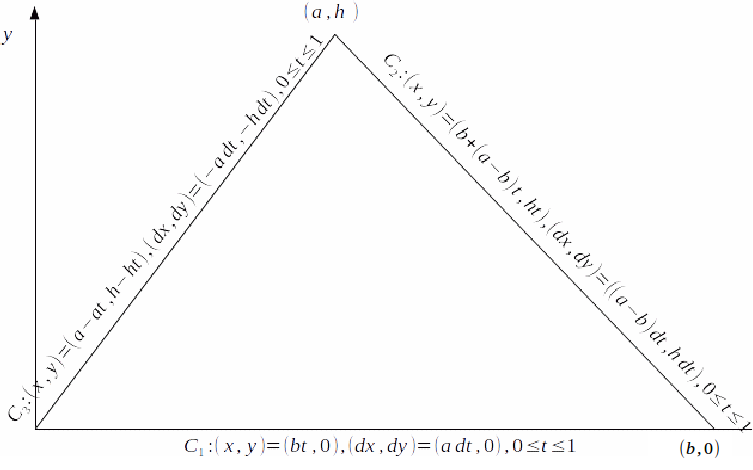
\[C_1\]
is \[(x,t)=(at, 0), \: (dx,dy) = (a dt, 0) , \: 0 \leq t \leq 1\]
The eqquation of
\[C_2\]
is \[(x,t) = (b+(a-b)t, ht), \: (dx, dy) =( (a-b) dt, h dt ), \: 0 \leq t \leq 1 \]
The eqquation of
\[C_3\]
is \[(x,t) = (a-at,h ht), \: (dx, dy) = (-a dt, -bdt), \: 0 \leq t \leq 1 \]
Because
\[y=dy=0\]
on \[C_1\]
the integral along \[C_1\]
does not contribution to the contouur integral.Take
\[P=0, \: Q=x\]
. Along \[C_2\]
\[\oint x \:dy =\int^1_0 (b+(a-b)t) h dt =[bht+(a-b)h \frac{t^2}{2} ]^1_0 =hb+(a-b) \frac{h}{2} =(a+b) \frac{h}{2}\]
Along
\[C_3\]
, \[\oint x \: dy =\int^1_0 (a-at) (-h) dt = [-aht + \frac{aht^2}{2}]^1_0 =-ah+ \frac{ah}{2} = - \frac{ah}{2} \]
The integral, and the area are the sum of thesed
\[\frac{bh}{2}\]
