Theorem
If
is a set, the smallest closed set containing
is the closure of
We can also write
where
is closed and
for each
Proof
Letbe a set and
such that
Suppose
and
Since eachis closed
is also closed and
is open.
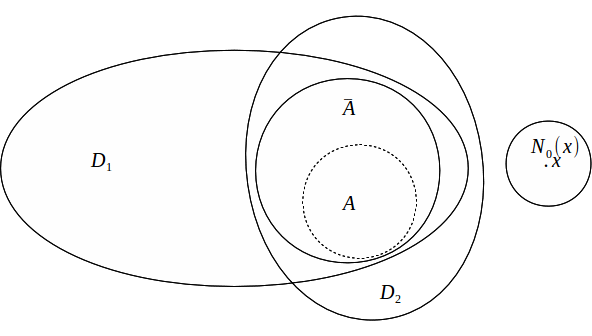
Hence x has a neighbourhood
of
such that
Now we must prove
Suppose
then there is a neighbourhoodof
such that
Therefore
is closed and contains
for some
Since