Proof of Euler's Equation

To prove Euler's equationfor a simple closed surface (homeomorphic to a sphere) start with a polygon drawn on the surface. Suppose the polygon has N vertices and hence N sides. The polygon has two faces - inside and outside the boundary so

Suppose we now join two vertices by a polygonal curve - shown as red lines with blue vertices below.

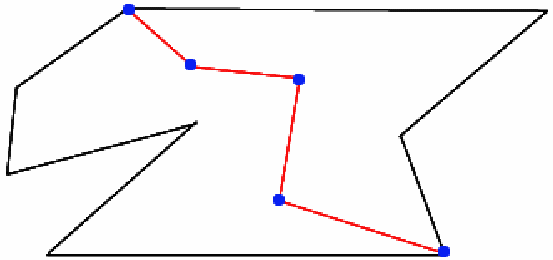
For a general curve of this type we addnew edges, n-1 new vertices qnd one new face. There are nowfaces,edges andvertices.

If instead we do not draw the polygonal curves between existing vertices, then we will create an additional two edges in addition to theedges along the polygonal curve we are adding, and two new vertices, where the new curve joins the existing polygon. In this case,and

This method can obviously be extended to any closed surface.