Proof That Every Metric Space is First Countable

Theorem

Every metric space is a first countable space.

Proof

A topological spaceis first countable if, for eacha countable setof open sets, each set containingexists with each open setcontainingcontains a point ofThat is,is first countable if and only if, at every pointa countable local base exists.

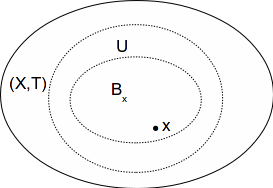
Letbe a metric space and letThe set of open balls

forms a countable local basis at

For every open setcontaininga ballexists.

Hence every metric space is a first countable space.