Proof That Every Separable Metric Space is Second Countable

Theorem

Ifis a separable metric space it is second countable.

Proof

Letbe a separable metric space. Let A be a countable dense subset ofso

Letbe the set of all open balls with centres inand with rational radii:

andare countable sets, hence so is

Takewhereis open inThere is an open ballsuch that

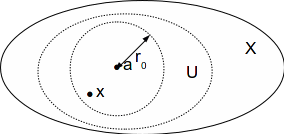
Sinceis dense inwe can findsuch that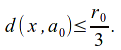

Letbe a rational number satisfying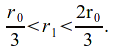

Then

Sinceandis countable,is a countable base for the given topology on