In Newtonian mechanics, the distance between simultaneous events events is a number that can be agreed on by all observers, and is equal to
In special relativity, observers do not agree on the distance or time intervals between events – in particular, events which are simultaneous from the point of view of one observer may not be simultaneous for another observer. A new definition of the distance between events is required. This definition is given by the spacetime interval
Two observers observing two events to have a difference in coordinate
and
wil obtain the same values for
and
this difference is labelled
and is called the invariant spacetime interval and can be positive, negative or zero. The distinction is important.
If
then the event occurring earliest may not be a possible cause of the later event. The distance between the two events is greater than can be covered by a light particle in a time
If
then the event occurring earliest may be a possible cause of the later event. The distance between the two events is equal to the distance that can be covered by a light particle in a time
If
then the event occurring earliest may be a possible cause of the later event. The distance between the two events is less than can be covered by a light particle in a time
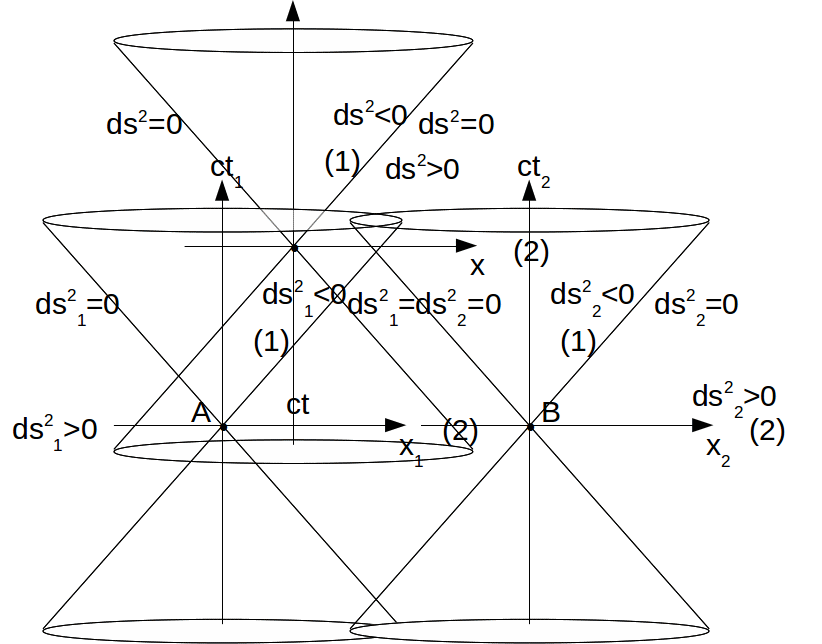
These different possibilities can be illustrated on a spacetime diagram.
The event at the cone vertex may be the cause of any event in region (1) in the diagram below, for which
but not in region (2) for which
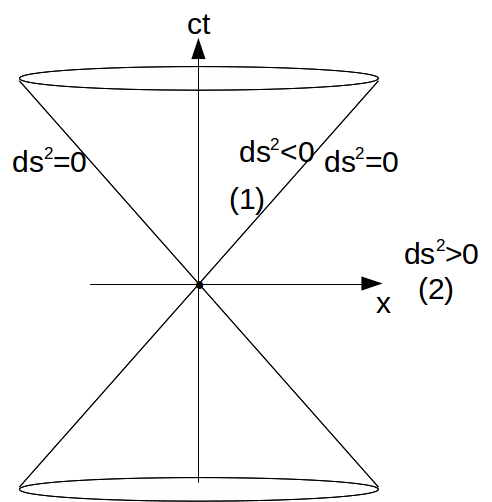
In region (2) different observers may not even agree about the order of events.
Similarly the event at the origin lies in the region
for event A below, so might have been caused by A, but not by event B, since it lies in the region
for event B.
