The usual methods of integrating – rearrangement, substitution, use of an identity of some form, or integration by parts – do not always work. If they do not work, it is often necessary to estimate the value of an integral. An estimation can usually be made using techniques of numerical integration. These techniques are usually derived by considering the area represented by the integral, since an integral of a function between two limits is just the area under the graph of the function between those limits.
Quite simple functions often cannot be integrated exactly. Some examples are given below.
![]()
![]()
The integrals do exist as numbers, since the functions![]() and
and![]() are well behaved and finite on
are well behaved and finite on![]() and
and![]() respectively. The integral of
respectively. The integral of![]() between
between![]() and
and![]() is shown below, indicated by the letter
is shown below, indicated by the letter![]() to represented the area under the graph and the value of the integral.
to represented the area under the graph and the value of the integral.
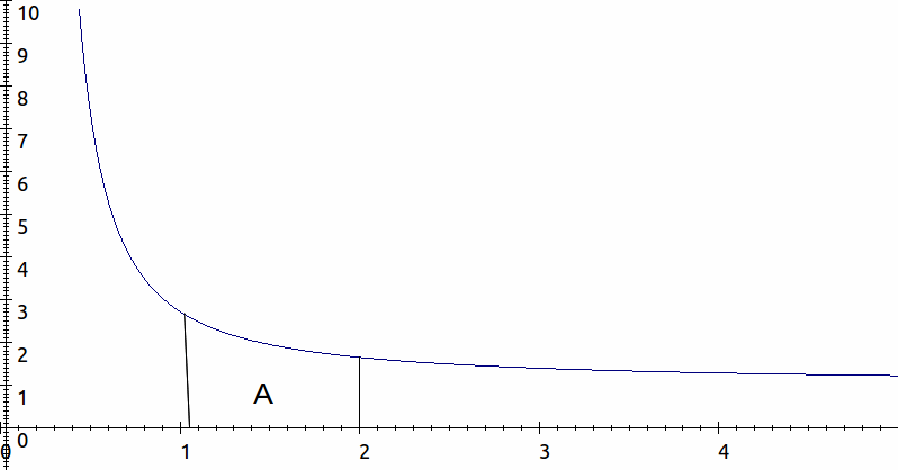
There are several ways of estimating the integral. All try to approximate the area![]() in different ways – with trapeziums in the Trapezium Rule, rectangles in the Mid Ordinate Rule, or the area under a series of sections of parabolas in Simpsons Rule.
in different ways – with trapeziums in the Trapezium Rule, rectangles in the Mid Ordinate Rule, or the area under a series of sections of parabolas in Simpsons Rule.
