Any expression of the form
is a difference of squares and factorises:
For example, if
then
and
(note we always take the positive square root) so
Sometimes we need to factorise with any common factors before we can use the difference of squares factorisation.
Now put
so that
and
The roots of the difference of squares
is the set of values of
for which
The roots are always equal in magnitude and opposite in sign. If an quadratic expression factorises into a difference of squares this is always the case. If we sketch this function, it is a quadratic graph with the line of symmetry
and minimum at
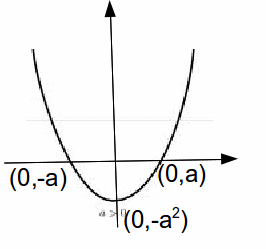
The roots of the difference of squares
are x=-a and x=a. If we sketch this function, it is a quadratic graph with the line of symmetryand minimum at
This is a 'sad' curve, as opposed to the 'happy' curve above.
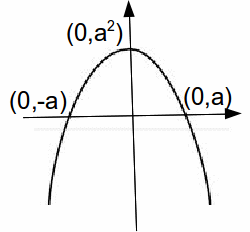
The above examples are quadratics, with
terms but this need not be the case.
