Group Actions
Let
be a set and let
be a group who elements act on the set
A left group action is a function
such that:
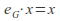for all
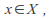where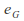
is the identity element in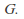
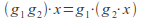for all
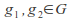and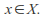
Right actions are similarly defined.
From these two axioms, it follows that for every
the function which maps
to
is a bijective map fromtoTherefore, one may alternatively and equivalently define a group action ofonas a group homomorphism fromto the set of all bijective maps fromto
If a group action
is given, we also say thatacts on the setoris a- set.
Every group
acts on itself in two natural ways: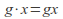
for all
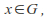or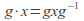
for all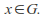
The symmetric group
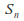and its subgroups act on the set
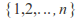by permuting its elements.The symmetry group of a polyhedron acts on the set of vertices of that polyhedron.
The symmetry group of any geometrical object acts on the set of points of that object.
The automorphism group of a vector space (or graph, or group, or ring...) acts on the vector space (or set of vertices of the graph, or group, or ring...).
The Matrix groups
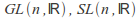and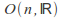
act on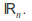
The Galois group and every subgroup of a field extension
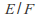acts on the bigger field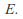
The additive group of the real numbers
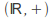acts on the phase space of systems in classical mechanics (and in more general dynamical systems): if
and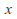
is in the phase space, then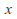describes a state of the system, and
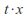is defined to be the state of the system
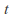seconds later if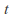is positive or
seconds ago if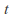is negative.
