Deprecated: Methods with the same name as their class will not be constructors in a future version of PHP; plgContentJComments has a deprecated constructor in /var/www/astarmathsandphysics/plugins/content/jcomments/jcomments.php on line 25 Call Stack: 0.0001 360856 1. {main}() /var/www/astarmathsandphysics/index.php:0 0.0557 1211056 2. Joomla\CMS\Application\SiteApplication->execute() /var/www/astarmathsandphysics/index.php:49 0.0557 1211056 3. Joomla\CMS\Application\SiteApplication->doExecute() /var/www/astarmathsandphysics/libraries/src/Application/CMSApplication.php:267 0.1284 4131640 4. Joomla\CMS\Application\SiteApplication->dispatch() /var/www/astarmathsandphysics/libraries/src/Application/SiteApplication.php:233 0.1298 4159400 5. Joomla\CMS\Component\ComponentHelper::renderComponent() /var/www/astarmathsandphysics/libraries/src/Application/SiteApplication.php:194 0.1306 4177112 6. Joomla\CMS\Component\ComponentHelper::executeComponent() /var/www/astarmathsandphysics/libraries/src/Component/ComponentHelper.php:356 0.1308 4207632 7. require_once('/var/www/astarmathsandphysics/components/com_content/content.php') /var/www/astarmathsandphysics/libraries/src/Component/ComponentHelper.php:381 0.1318 4230352 8. ContentController->execute() /var/www/astarmathsandphysics/components/com_content/content.php:42 0.1318 4230352 9. ContentController->display() /var/www/astarmathsandphysics/libraries/src/MVC/Controller/BaseController.php:710 0.1893 4912128 10. ContentController->display() /var/www/astarmathsandphysics/components/com_content/controller.php:113 0.1930 5104280 11. Joomla\CMS\Cache\Controller\ViewController->get() /var/www/astarmathsandphysics/libraries/src/MVC/Controller/BaseController.php:663 0.1935 5125208 12. ContentViewArticle->display() /var/www/astarmathsandphysics/libraries/src/Cache/Controller/ViewController.php:102 0.2062 5341776 13. Joomla\CMS\Plugin\PluginHelper::importPlugin() /var/www/astarmathsandphysics/components/com_content/views/article/view.html.php:189 0.2063 5342032 14. Joomla\CMS\Plugin\PluginHelper::import() /var/www/astarmathsandphysics/libraries/src/Plugin/PluginHelper.php:182 Deprecated: Methods with the same name as their class will not be constructors in a future version of PHP; JCommentsACL has a deprecated constructor in /var/www/astarmathsandphysics/components/com_jcomments/classes/acl.php on line 17 Call Stack: 0.0001 360856 1. {main}() /var/www/astarmathsandphysics/index.php:0 0.0557 1211056 2. Joomla\CMS\Application\SiteApplication->execute() /var/www/astarmathsandphysics/index.php:49 0.0557 1211056 3. Joomla\CMS\Application\SiteApplication->doExecute() /var/www/astarmathsandphysics/libraries/src/Application/CMSApplication.php:267 0.1284 4131640 4. Joomla\CMS\Application\SiteApplication->dispatch() /var/www/astarmathsandphysics/libraries/src/Application/SiteApplication.php:233 0.1298 4159400 5. Joomla\CMS\Component\ComponentHelper::renderComponent() /var/www/astarmathsandphysics/libraries/src/Application/SiteApplication.php:194 0.1306 4177112 6. Joomla\CMS\Component\ComponentHelper::executeComponent() /var/www/astarmathsandphysics/libraries/src/Component/ComponentHelper.php:356 0.1308 4207632 7. require_once('/var/www/astarmathsandphysics/components/com_content/content.php') /var/www/astarmathsandphysics/libraries/src/Component/ComponentHelper.php:381 0.1318 4230352 8. ContentController->execute() /var/www/astarmathsandphysics/components/com_content/content.php:42 0.1318 4230352 9. ContentController->display() /var/www/astarmathsandphysics/libraries/src/MVC/Controller/BaseController.php:710 0.1893 4912128 10. ContentController->display() /var/www/astarmathsandphysics/components/com_content/controller.php:113 0.1930 5104280 11. Joomla\CMS\Cache\Controller\ViewController->get() /var/www/astarmathsandphysics/libraries/src/MVC/Controller/BaseController.php:663 0.1935 5125208 12. ContentViewArticle->display() /var/www/astarmathsandphysics/libraries/src/Cache/Controller/ViewController.php:102 0.3971 13764392 13. JEventDispatcher->trigger() /var/www/astarmathsandphysics/components/com_content/views/article/view.html.php:199 0.3974 13764792 14. plgContentJComments->update() /var/www/astarmathsandphysics/libraries/joomla/event/dispatcher.php:160 0.3974 13764792 15. plgContentJComments->onContentAfterDisplay() /var/www/astarmathsandphysics/libraries/joomla/event/event.php:70 0.3976 13773040 16. plgContentJComments->onAfterDisplayContent() /var/www/astarmathsandphysics/plugins/content/jcomments/jcomments.php:339 0.3980 13774776 17. JComments::show() /var/www/astarmathsandphysics/plugins/content/jcomments/jcomments.php:282 0.3990 13807824 18. JCommentsFactory::getACL() /var/www/astarmathsandphysics/components/com_jcomments/jcomments.php:188 0.3990 13808224 19. spl_autoload_call() /var/www/astarmathsandphysics/components/com_jcomments/classes/factory.php:274 0.3991 13808304 20. JLoader::load() /var/www/astarmathsandphysics/components/com_jcomments/classes/factory.php:274

The g Over h Rule for Evaluating Residues

Theorem

Letwhere the functionsandare analytic at the pointand then

Proof:

In order to prove the theorem we first need to show that an if an analytic functionhas a singularity atthenproviding this limit exists.

To do this letIfthenhas a removable singularity atIf then f has a simple pole atand

(Note that we can expandin a Laurent series aboutobtaining

so that

then)

Now putthen(since ), then

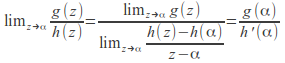
The theorem is proved.

Example: Evaluate the residue ofat

atandatso therule gives

Add comment

Security code
Refresh