A Hausdorff or
space is a topological space in which distinct points have disjoint neighbourhoods, implying that any two points are part of disjoint open sets.
are Hausdorff with the usual topology because if
then
so each of
can be placed inside open balls of radius
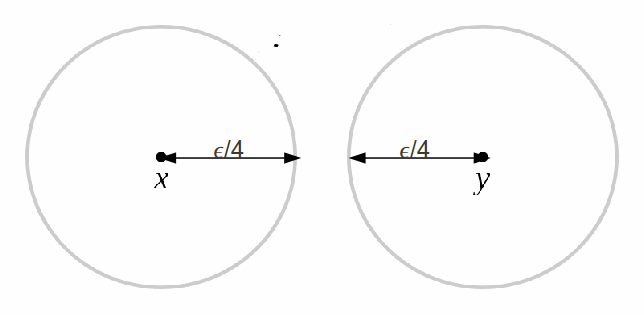
The Hausdorff condition implies the uniqueness of limits of functions, since if
is continuous and
a sequence convergent to x so that
and
are subsequences ofthen for
given there exists
such that
implies
Take
Sinceis convergent and f is continuous there exists
such that
and similarly
implies
Hence
Hence
This is a contradiction hence limits of functions are unique. Since
is also a sequence, limits of sequences are unique.